 |
 |
 |
 |
 |
|
Each year, estimates of future income and expenditures of the Old-Age and Survivors Insurance and Disability Insurance (OASDI) program are presented to the Congress in the Annual Report of the Board of Trustees. These estimates illustrate possible scenarios of the future financial position of the OASDI program, under present law, and thus are valuable in the policy making process for the program.
To produce these financial estimates, projections of the population in the Social Security coverage area are needed. One of the essential components of population projections is a projection of mortality, which is the subject of this study. For the 2002 Trustees Report, three separate projections - intermediate, low cost, high cost - were prepared. These projections are based on three different sets of assumptions about future death rates. The intermediate projections are thought to be the most likely to occur among the three sets. All mortality projections presented in this study are from the intermediate projections of the 2002 Annual Report of the OASDI Board of Trustees. These projections were also used in estimating the future financial status of the Hospital Insurance (HI) and Supplementary Medical Insurance (SMI) programs as described in the 2002 Annual Report of the Medicare Board of Trustees.
Mortality rates are presented in this study in the context of life tables, which are commonly used by actuaries and demographers. Tables on both period and cohort bases are included. These tables supersede those published in Actuarial Study Number 107, which were used in the preparation of the 1992 Annual Reports.
A life table is a concise way of showing the probabilities of a member of a particular population living to or dying at a particular age. In this study, the life tables are used to examine the mortality changes in the Social Security population over time.
An ideal representation of human mortality would provide a measure of the rate of death occurring at specified ages over specified periods of time. In the past, analytical methods (such as the Gompertz, Makeham, or logistic curves) satisfied this criterion approximately over a broad range of ages. However, as actual data has become more abundant and more reliable, the use of approximate analytical methods have become less necessary and acceptable. Today, mortality is most commonly represented in the form of a life table, which gives probabilities of death within one year at each exact integral age. These probabilities are generally based on tabulations of deaths in a given population and estimates of the size of that population. All functions in the life table can be generated from the qx, where qx is the probability of death within a year of a person aged x. Although a life table does not give mortality at non-integral ages or for non-integral durations, as can be obtained from a mathematical formula, acceptable methods for estimating such values are well known.
Two basic types of life tables are presented in this study, period-based tables and cohort-based tables. Each type of table can be constructed either based on actual population data or on expected future experience.
A period life table is based on, or represents, the mortality experience of an entire population during a relatively short period of time, usually one to three years. Life tables based directly on population data are generally constructed as period life tables because death and population data are most readily available on a time period basis. Such tables are useful in analyzing changes in the mortality experienced by a population through time. If the experience study is limited to short periods of time, the resulting rates will be more uniformly representative of the entire period. This study presents period life tables by sex for decennial years 1900 through 1990 based on United States and Medicare data, and for decennial years 2000 through 2100 reflecting projected mortality.
A cohort, or generation, life table is based on, or represents, mortality experience over the entire lifetime of a cohort of persons born during a relatively short period of time, usually one year. Cohort life tables based directly on population experience data are relatively rare, because of the need for data of consistent quality over a very long period of time. Cohort tables can, however, be readily produced, reflecting mortality rates from a series of period tables for past years, projections of future mortality, or a combination of the two. Such tables are superior to period tables for the purpose of projecting a population into the future when mortality is expected to change over time, and for analyzing the generational trends in mortality. This study presents cohort life tables by sex for births in decennial years 1900 through 2000, reflecting the mortality experience and projections described above.
A life table treats the mortality experience upon which it is based as though it represents the experience of a single birth cohort consisting of 100,000 births who experience, at each age x of their lives, the probability of death, denoted qx, shown in the table. The entry lx in the life table shows the number of survivors of that birth cohort at each succeeding exact integral age. Another entry, dx, shows the number of deaths that would occur between succeeding exact integral ages among members of the cohort. The entry denoted Lx gives the number of person-years lived between consecutive exact integral ages x and x+1 and Tx gives the total number of person-years lived beyond each exact integral age x, by all members of the cohort. The final entry in the life table, 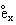 , represents the average number of years of life remaining for members of the cohort still alive at exact integral age x, and is called the life expectancy.
, represents the average number of years of life remaining for members of the cohort still alive at exact integral age x, and is called the life expectancy.
The lx entry in the life table is also useful for determining the age corresponding to a specified survival rate from birth, which is defined as the age at which the ratio of lx to 100,000 is equal to a specified value between 0 and 1.
A stationary population is what would result if for each past and future year:
A population with these characteristics would have a constant number of persons from year to year (in fact, at any time) both in their total number and in their number at each age. These numbers of persons, by age last birthday, are provided in the life table as the Lx values. The lx entry is interpreted as the number of persons who attain each exact integral age during any year, and dx is the number of persons who die at each age last birthday during any year. The entry Tx represents the number of persons who are alive at age last birthday x or older, at any time.
Annual tabulations of numbers of deaths by age and sex are made by the National Center for Health Statistics (NCHS) based on information supplied by States in the Death Registration Area, and are published in the volumes of Vital Statistics of the United States. These are now available on the web at www.cdc.gov/nchs. Deaths are provided by five year age groups for ages 5 through 84, in total for ages 85 and older, and by single-year and smaller age intervals for ages 4 and under. One requirement for admission to the Death Registration Area, which since 1933 has included all the States, the District of Columbia and the independent registration area of New York City, was a demonstration of ninety percent completeness of registration. Because incentives for filing a death certificate are so strong (obtaining burial permits, collecting insurance benefits, settling estates, etc.) and because every State has adopted laws that require the registration of deaths, it is believed that errors of under registration of deaths are insignificant for the nation as a whole. Errors of misstatement of age on the death certificate, however, may very well cause distortion in the distribution of numbers of deaths by age group.
Annual estimates of the U.S. resident population by single year of age and sex are made by the Census Bureau and are published in Current Population Reports Series P-25. The most recent population information is available and updated regularly on the Census Bureau web site at www.census.gov. These estimates are affected by both undercount and misclassification in the decennial census. These errors, which may either offset or compound, are usually considered together as net undercount. Postcensal estimates are made by the "inflation-deflation" method which inflates the last previous census-level population by net undercount, carries the inflated population forward according to the births and deaths tabulated in the Vital Statistics, adjusts the population by estimated net immigration, and then deflates by net undercount. Thus, the postcensal population estimates are affected by errors in the Vital Statistics and the effect tends to accumulate as the elapsed time from the last previous census increases. When results of the following census become available, the postcensal estimates are revised, and are then called intercensal estimates, thus removing much of the effect of errors in Vital Statistics and in net immigration estimates.
Central death rates calculated by comparing numbers of deaths tabulated by the National Center for Health Statistics to the mid-year population estimated by the Census Bureau are affected by the errors from both sources, which may either offset or combine. Further, errors of noncomparability of numerator and denominator may also be introduced. Although efforts are made to minimize errors of noncomparability (by excluding armed forces overseas from the population estimates, for example), complete comparability is intrinsically impossible.
The errors of noncomparability can be eliminated if the numbers of deaths and the population are drawn from the same source. This approach, however, generally involves so large a reduction in the size of the population being observed, that more random error is introduced than noncomparability error is eliminated. One source of data on aged persons which is not subject to errors of noncomparability and yet does permit a very large number of observations, is Medicare program enrollment. Also, this source involves fewer errors of misstatement of age, because most of the data relate to individuals who have had to prove their date of birth to become entitled to benefits.
An error analogous to net undercount does appear to be present in the Medicare data, although the error is believed to have an insignificant effect on calculated death rates, except for the very aged (beginning at roughly age 95). This error stems from the presence in the data of "phantom records" which may have arisen because the person was registered in the program more than once, or because information about a person was miscoded when he registered, or because the person's death was not reported. Such phantom records are not of much concern to cost-conscious program administrators, however, because the Medicare program only pays benefits when bills for covered services rendered are submitted.
In an effort to reduce the number of phantom records, the Medicare based death rates calculated for years after 1987 were limited to the records of those Medicare participants that were also eligible for Social Security or Railroad retirement monthly income benefits, or who were government employees or retirees with enough Medicare qualifying government employment. This limitation eliminated approximately three percent of the Medicare records.
Data needed in order to project central death rates by cause of death were obtained from Vital Statistics tabulations for years since 1979. For the years 1979-1998, adjustments were made to the distribution of the numbers of deaths by cause. The adjustments were needed in order to reflect the revision in the cause of death coding that occurred in 1999, making the data for the years 1979-1998 more comparable with the coding used for the years 1999 and later. The adjustments were based on comparability ratios published by the National Center for Health Statistics.
For the years 1900-1967, age-sex specific central death rates were calculated from NCHS Vital Statistics tabulations of deaths and Census estimates of populations. For the period 1968-1999 those same two sources were used for ages under 65, but records of the Medicare program were used to calculate rates for ages 65 and over. The numbers of deaths by cause from Vital Statistics tabulations were used to distribute the age-sex specific rates into age-sex-cause specific rates for the years 1979-1999.
Populations in some five-year age groups for some years were estimated from published figures for broader age groups. Death Registration States' populations during 1900-1932 for five-year age groups, 5-9 through 70-74, were estimated from the ten-year age groups, 5-14 through 65-74, by assuming that the distributions of five-year age groups within ten-year age groups were as published for the United States resident population from the Census Bureau. Death Registration States' populations during 1900-1932, and United States population during 1933-1939 for the age group 75-84, were distributed between the 75-79 and 80-84 age groups by using linear interpolation of the age distributions from the Decennial Census enumerations. Death Registration States' populations during years 1900-1932 and United States population during years 1933-1967 for age group 85-89, 90-94, and 95 and over were estimated by distributing the group 85 and over using NCHS tabulated deaths for each year and Medicare data. The split of the conterminous United States populations aged 0-4 into age groups 0 and 1-4 for the years 1950-1959 was estimated from the group 0-4 by assuming the same distribution as in the United States, Alaska, and Hawaii combined. For 1959, deaths occurring in Alaska were excluded from total deaths, so that the population of the conterminous United States could be used to calculate the death rates. For all years, deaths tabulated at "age unstated" were prorated across the tabulated age groups.